Division Algebra Dimension
Any linearly independent set of elements of V is a subset of a basis. Viewed 122 times 4 3 begingroup I want to understand about existence of some non-commutative division algebras over mathbbQ of dimension.
Frobenius Theorem Bresar Theorem 1 4 Physics Forums
In 1958 three mathematicians proved a decades old suspicion any division algebra must have dimensions one real numbers two complexnumbers four quaternions and eight octonions.
Division algebra dimension. Yellow objects 6 of more two of which are identical. The double sign causes the category of all real division algebras of a fixed dimension n1 to decompose into four blocks. Objects different shades of yellow and green.
In simpler terms it is the measurement of the length width and height of anything. That is the D-module isomorphism class of V is determined by the dimension of V. For each n2f248g the double sign causes the category D n of all n-dimensional real division algebras to decompose into four.
Then W is finitely-generated and there is a complementary submodule W0. We can now divide the Cli ord algebra Clpq into even and odd elements. Active 2 years 6 months ago.
One-dimensional or 1D Two-dimensional or 2D Three-dimensional or 3D For example. 117 Division Website opens new window. Let A be a Euclidean Hurwitz algebra and let M n A be the algebra of n-by-n matrices over A.
For any real division algebra Aof nite dimension greater than one the signs of the determinants of left multiplication and right multiplication by an element a2Anf0gare shown to form an invariant of A called its double sign. We show that if D is a division subalgebra of QA of GK dimension at least 2 then QA is finite dimensional as a left D-vector space. So N can only be 1 2 4 or 8.
George Parker III School Number. Let A be a finitely generated K-algebra that is a domain of GK dimension less than 3 and let QA denote the quotient division algebra of A. Frobenius proved in 1877 that the only division algebras over R of finite dimension are a R itself of dimension 1 b the complex numbers C a field of dimension 2 over R and c the quaternions H a skew field of dimension 4 over R.
Division is the critical part a number system in which we can divide is called division algebra. If C F the division algebra A is called a central division algebra. Any object or surroundings or space can be.
Crayons yellow and green. The even components of the Cli ord algebra make up the even or second Cli ord algebra. For any real division algebra A of finite dimension greater than one the signs of the determinants of left multiplication and right multiplication by a non-zero element are shown to form an.
This means that if you use Bbb R-coefficients you still get a division algebra as you know but you also get a division algebra if you allow Bbb Q_2-coefficients instead. Middle 06-08 School Phone. There is a unique integer n the D-dimension of V so that V Dn as a D-module.
Now to answer your original question it remains to notice that the dimensions of V as a left and a right D-module coincide since both are equal to dim_KVdim_KD. Newport News City Public Schools Division Number. Applications to Jordan algebras.
400 Atkinson Way Newport News VA 23608 Principal. Objects hard and soft at least 1 per student. For any real division algebra A of finite dimension greater than one the signs of the determinants of left multiplication and right multiplication by a non-zero element are shown to form an invariant of A called its double sign.
Containers one of each color per center including red blue yellow green and transparent. Ask Question Asked 2 years 6 months ago. So the dim_D Votimes_D W ndim_D V where dim_D denotes the dimension of left D-modules.
A division algebra over the real numbers R is a division ring that has the reals as a subfield. The second Cli ord algebra is the algebra of eigenvectors of the grade involution with eigenvalue one and is written as 213 Clpq M 0 M 2. Mary Passage Middle General school information.
The regular quaternion division algebra Bbb H that you know is ramified only at infty and 2. If N 6 the dimension is 4 which does not divide 6. Division algebra over rationals of dimension 9.
It is a unital nonassociative algebra with an involution. Finite-dimensional central associative division algebras over F may be identified up to an isomorphism with the elements of the Brauer group B F of the field F. Objects red blue yellow green and some other colors.
In particular this dimension is N so N is less than or equal to 8. Let W be a submodule of V.
06 Simplifying Algebraic Expressions That Involve Division Part 1 Youtube
Find Dimension Of Solid Box For Given Volume Polynomial Equations Application Youtube
Finite Dimensional Division Algebras Over Fields Nathan Jacobson Springer
Dividing Polynomials College Algebra
Linear Algebra And Higher Dimensions Science4all
Dividing Polynomials College Algebra
Linear Algebra And Higher Dimensions Science4all
Area Model Algebra Polynomials Factors Products Phet Interactive Simulations
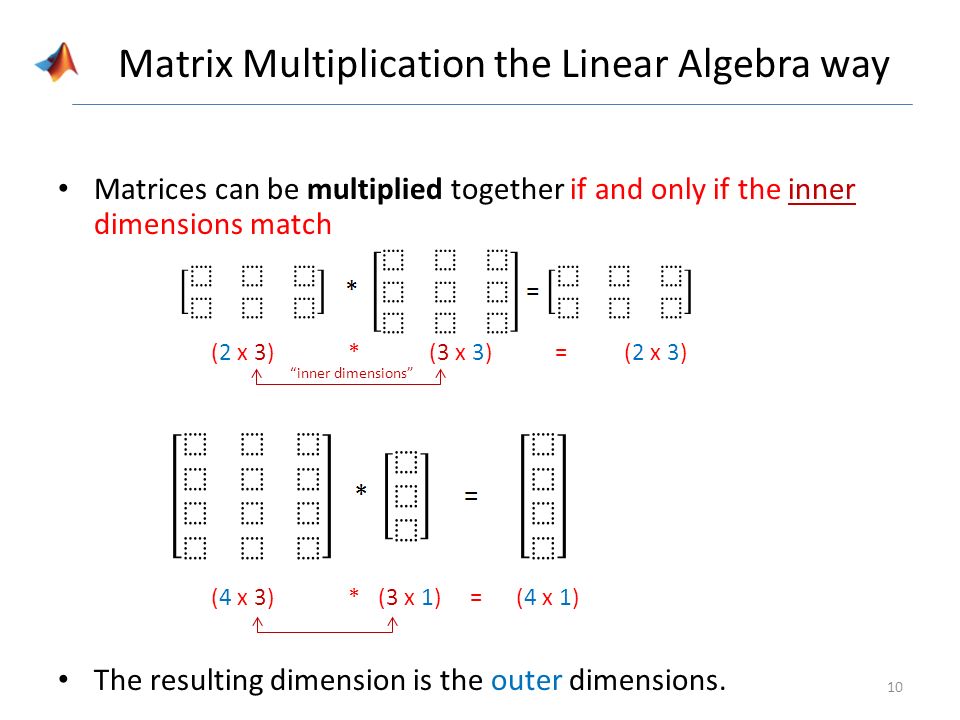
Array Math Ppt Video Online Download
Algebra Ii 13 6c Dividing Matrices By Using Inverses Youtube
Find Missing Dimension Write Expression Intro Algebra 102 Youtube
Https Math Usask Ca Bremner Research Publications Beamer 20 20hdax Pdf
Linear Algebra 6 Rank Basis Dimension By Adam Dhalla Medium
Normed Division Algebra In Nlab
Algebra Ii Honors Test Review 6 1 To 6 4
Complex Dimensions And Dimensional Analysis Nexus Wiki
Algebra Paper Retracted Because Of Questions About The Integrity Of The Mathematics Retraction Watch
